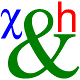
{ Practical astronomy | Astronomy | Stars and star clusters }

Stars and star clusters

The principal objects we see in a clear, moonless night are stars, tiny points of light, some bright, many dim, and some with different colour than others.

The daily motion of the stars from dusk to dawn is merely a mirror of the rotation of the Earth and at first sight similar to the motion of the Sun from dawn to dusk. The annual change of the stars through the seasons is a different matter. Because the Sun moves around the sky over the year, the star patterns opposite the Sun – those visible at night – also change over the year.

If we sort stars into patterns that we call constellations, then we can identify these and spot the change of the seasons. This is very important for hunters, gatherers and farmers, unless they have a calendar app on their mobile phone to tell them the time of year.

Field stars and constellations

The constellation of Orion.

The constellation of Orion in a modern star chart (Sinnott and Flenberg 2011a).

The constellation of Orion in the Uranometria star atlas (Bayer 1603a; image courtesy USNO and Wikimedia).

The constellations of Cassiopeia and Perseus.

The constellations in use today go back to the ancient Greeks, who transplanted their mythology into the heavens. When European explorers gained access to the southern hemisphere they added further constellations to fill the sky that had been unseen by Europeans until then. The list of 88 constellations, with Latin names and three-letter abbreviations, was made official by the International Astronomical Union (IAU) in 1921. Even then, the constellations were patterns of bright'ish stars with imagined lines connecting them. Older star catalogues also show more lifelike depictions of the persons or objects (Greek gods, scientific instruments, etc.) represented by the constellations. In 1928 the IAU also drew boundary lines between constellations, so that any celestial coordinate is now unambiguously part of exactly one of the 88 constellations.

Some constellations are easy to spot, many difficult. The IAU has given all a Latin name, a genitive form of its name that is used in conjunction with star names, and a three-letter abbreviation of the genitive. Among the easy constellations are

Orion, Orionis, Ori

The main body of the constellation combines the three stars of the hunter's belt with four very bright corner stars representing the shoulders and feet. This main body is about 20° in size. The belt stars point, moving left and down, to the brightest star visible from Europe, Sirius. Moving up and right, the belt stars point to Aldebaran, a bright red star in Taurus in front of the open star cluster of the Hyades.
The first image was taken with a stationary dSLR and 30 s exposure (f/5.6, 1600 ISO); note the short star trails. Second is a modern star chart. This includes the star-to-star lines that help memorise the star pattern. They also show the modern boundaries to neighbouring constellations. Third is the constellation from the Uranometria star atlas of 1603, giving an idea of how the ancient Greeks might have imagined it.

Ursa Major, Ursae Majoris, UMa

The main body of seven, quite bright stars that form the shape of a plough or saucepan. In the full constellation this main part forms the hip and tail of the (female) bear. The main body is about 30° long, the entire constellation is about 40° or 50° in size. In modern times, the constellation is often used for approximate navigation: The two brightest and rightmost stars of the saucepan (away from the handle) point approximately to the pole star and therefore indicate the North direction on Earth. Ursa Major is also known for the double star Mizar/Alcor, in the handle of the saucepan; this can be resolved with the naked eye.

Cassiopeia, Cassiopeiae, Cas

The main star pattern is relatively compact at only 15°. The constellation is also known as "the W", as this letter can be read into the pattern at the correct orientation and if one ignores a sixth star of similar brightness. These stars are very bright. The star pattern, including the sixth star, depict the chair of Queen Cassiopeia, wife of Cepheus and mother of Andromeda.
The image was taken with a stationary dSLR and 5 min exposure (f/3.5, 100 ISO). Cassiopeia is above, Perseus below.

Lyra, Lyrae, Lyr

This is a tiny constellation of only 10° size. However, it includes the star Vega, the brightest star in the northern hemisphere. (Sirius is brighter and visible from much of the terrestrial northern hemisphere, but Sirius is in the celestial southern hemisphere.) Further, there is the double star ε Lyrae. This can be resolved with the naked eye, providing perfect eyesight. These two stars are physically bound, and each is in fact a close double star that requires 150 or 200 mm aperture to resolve.

Very many stars have names that involve the constellation. For example, the brightest star in Ursa Major is also known as α Ursae Majoris, in essence "the brightest star of Ursa Major". This is then abbreviated to α UMa. The use of lower-case Greek letters goes back to the Uranometria (Bayer 1603a). In most constellations the Greek alphabet orders the stars from brightest to fainter stars. Flamsteed in 1712 introduced a similar, if different, scheme. This extends to fainter stars, which by then had been discovered with telescopes. Here the stars in a constellation are numbered from right to left (by increasing right ascension).

More modern object names that involve the constellation name tend to use not the genitive, but the name itself. For example, Cygnus A is a bright radio source in the area of the constellation of Cygnus. The genitive would have been Cygni, as in "Deneb is also called α Cygni".

The Milky Way galaxy

The band of the Milky Way.

A spiral galaxy.

The constellations are constructed from the brightest stars. There are more "field stars", of which a few thousand are visible to the naked eye. Telescopes reveal fainter field stars. Then there is the Milky Way. From a good dark sky site, we can see the Milky Way as a band of dim star light, the sum of light from hundreds of thousands of stars that are individually too dim and far away to be seen with the naked eye. The band forms a great circle all around the celestial sphere.

The first image shows this band of light, the view here stretching from Scorpius on the far left via Centaurus to the Coal Sack dark cloud and Crux (the Southern Cross) just top right from it. The bright patch on the right margin is η Carinae.

The band of light is in many parts split in two. This is because within the stellar disc there is a thinner layer of interstellar gas and dust. Within that narrower region, the dust limits our few to a few kpc distance and hides stars that lie beyond.

The second image shows an imaginary view of the Milky Way from outside. Actually, it is an image of a similar neighbour galaxy. We see a bright band all around our sky, because we are actually in the thin stellar disc. The image does not demonstrate how thin: the stellar disc has a radius of perhaps 10 kpc but only a thickness of 1 kpc.

The word galaxy stems from the Greek word for milk. As such the Galaxy, with capitalisation, is just another term to refer to the (our) Milky Way. Without capitalisation we use the term galaxy for any such object, our own Milky Way that we are part of, but also other, external galaxies.

Flux, magnitude, size, distance

The stars are evidently too far away to resolve their surface. We see only their integrated flux S, which is essentially its surface brightness added up over the earth-facing hemisphere of its surface.

While radio astronomers prefer to work with source fluxes and managed to calibrate them in terms of of electric power received per antenna area and receiver bandwidth, visible-light astronomers use a much older and more intuitive measure. In fact the use of magnitude for stellar brightness goes back to Ptolemy (+137, 137 AD), but Hipparchus, around −130 (around 130 BC), used a similar scale in his star catalogue (Miles 2007). The system was put on a modern mathematical footing by Pogson (1856), such that every 5 mag difference corresponds to a factor 100 in source flux. For visual magnitudes we now have (UKIRT 1998):

S = 3540 · 10^−0.4·V Jy

V = −2.5 · lg(S / 3540 Jy)

where Jansky (Jy) is a flux measure originally used by radio astronomers and rooted in the SI system of physical units. It is defined in terms of energy received per square metre of antenna surface, per Hz of receiver bandwidth and per second of integration time.

1 Jy = 10⁻²⁶ · W/m² / Hz

We note that the flux and magnitude of a star refer to a particular wavelength or colour of light and a particular filter transmission. Say, the human eye is most sensitive near 500 or 550 nm wavelength and its sensitivity drops to nothing around 400 nm on the blue side and 700 nm on the red side. By visual magnitude we these days mean a particular green filter called a "Johnson V" filter.

Mathematically, it turns out that the very brightest stars are too bright for "magnitude one". Rather, they are 0 mag or even −1 mag. The Sun, because of its proximity, is even at −27 mag. And, of course, large telescopes now observe stars too faint for the naked eye, with magnitudes +15 or +20. The magnitude scale is good news and it is bad news. The good news is (i) that its logarithmic scale is much more adequate when such vast ranges of flux values occur and (ii) that the numbers then become much more manageable and memorable. The bad news is that the magnitude scale runs backwards: A higher flux or brighter star means a smaller magnitude; a larger magnitude implies a dimmer and less important star. When someone speaks of "greater magnitude" do they man a dimmer or a brighter magnitude? And in software, the programmer has to take care not to forget the minus sign in the relationship between magnitude and anything describing brightness in a modern way.

The fact that there are few bright and many dimmer stars is largely, but not entirely, due to their different distances. A star at twice the distance appears four times fainter even if it has the same actual, inherent brightness or flux. This leads to the distinction between apparent and absolute magnitude. The absolute magnitude is usually just a tool used when comparing stars that are at different distances from us.

1991.25 2000.0  Hipparcos catalogue.
   RA        Dec     pi    V    B-V  BaFlVar name common name
--------- --------- ---- ----- ----- ------------ -----------------
101.28854 -16.71314  379 -1.44  0.01    alpha CMa Sirius
 95.98788 -52.69572   10 -0.62  0.16    alpha Car Canopus
213.91811  19.18727   89 -0.05  1.24    alpha Boo Arcturus
219.92041 -60.83515  742 -0.01  0.71  alpha^1 Cen Rigel Kentaurus A
279.23411  38.78299  129  0.03 -0.00    alpha Lyr Vega
 79.17207  45.99903   77  0.08  0.80    alpha Aur Capella
 78.63446  -8.20164    4  0.18 -0.03     beta Ori Rigel
114.82724   5.22751  286  0.40  0.43    alpha CMi Procyon
 24.42813 -57.23666   23  0.45 -0.16    alpha Eri Achernar
 88.79287   7.40704    8  0.45  1.50    alpha Ori Betelgeuse
210.95602 -60.37298    6  0.61 -0.23     beta Cen Hadar
297.69451   8.86738  194  0.76  0.22    alpha Aql Altair

Astronomers use a different distance unit for the stars, the parsec. This has to do with how they measure stellar distances properly. They observe the same star at opposite times of the year. The movement of the Earth around the Sun places the telescope 300 Gm away from the first position, and this causes a small shift, or parallax, in the position of the star. If this parallax is two arcsecond (one arcsecond for a shift of one astronomical unit, the Sun-Earth distance), we speak of a distance of one parsec or 1 pc. Beware of another inversion of scales: A star further away, say 10 pc, has a smaller parallax, namely π=0.1".

r / pc = 1 / π

1 pc = 3.0857 · 10¹³ km ≈ 3.26 ly

In this unit our estimated neighbour distance is about 1.3 pc.

1991.25 2000.0  Hipparcos catalogue.
   RA        Dec     pi    V    B-V  BaFlVar name common name
--------- --------- ---- ----- ----- ------------ -----------------
217.44895 -62.68135  772 11.01  1.81  alpha C Cen Proxima Centauri
219.92041 -60.83515  742 -0.01  0.71  alpha^1 Cen Rigel Kentaurus A
219.91413 -60.83947  742  1.35  0.90  alpha^2 Cen Rigel Kentaurus B
269.45402   4.66829  549  9.54  1.57              Barnard's Star
165.83588  35.98146  392  7.49  1.50              B+36  2147  
101.28854 -16.71314  379 -1.44  0.01    alpha CMa Sirius
282.45398 -23.83576  336 10.37  1.51    V1216 Sgr
 53.23509  -9.45831  311  3.72  0.88  epsilon Eri
346.44652 -35.85630  304  7.35  1.48              C-36 15693  
176.93351   0.80753  300 11.12  1.75       FI Vir
316.71181  38.74149  287  5.20  1.07     61 A Cyg
114.82724   5.22751  286  0.40  0.43    alpha CMi Procyon
316.71747  38.73441  285  6.05  1.31     61 B Cyg
280.70212  59.62236  284  9.70  1.56              HIP 91772
  4.58559  44.02196  280  8.09  1.56       GX And

This table of nearest stars tells us two other, very important things:

1. There are a lot of stars in multiple systems. In our sample of 12 systems, one is at least double, another at least triple. And there are probably more multiple stars in this list, where we have catalogued only the brightest of two or three.
2. The brightness range is vast. Stars are inherently not of the same brightness at all. There is about 10 mag difference, or a factor 10000 in flux between the brightest and the faintest stars (when seen from the same distance).

Black body radiation

The definition of a star (strictly, of a main sequence star with solar metallicity) is that it is a ball of gas of 75% hydrogen, 24% helium and 2% heavier elements, which at its centre fuses hydrogen into more helium. The fusion process releases energy, this heats the star, including its surface. The surface then emits light according to its size and temperature.

There must exist a balance between the energy from fusion and the light emitted into space. At its surface the star is opaque so that the rules of the black body apply: The hotter the surface of the star, the higher the surface brightness, but also the shorter the average wavelength of the emission (the more blue and less red is the overall impression of the star). Max Planck in 1914 famously solved the question of how bright a black body is at any wavelength, given its temperature. To do this he had to invent the fundamental constant h that turned light of any wavelength λ or frequency ν=c/λ into quanta of energy hν, thus introducing the photon as elementary particle and ringing in the age of quantum physics. We have (Kneubühl 1982a, p.426):

Planck spectra B_λ.

B_ν = [2 h ν³ / c²] / [exp(hν/kT) − 1]

B_λ = [2 h c² / λ⁵] / [exp(hc/λkT) − 1]

Mathematically, there are two versions. One gives the energy per frequency interval and the other per wavelength interval. The principal wisdom from either is the same, but when you crunch numbers you have to be wary of using the right version. The graph shows B_λ for the four black-body temperatures of 10 K, 100 K, 1000 K and 10000 K. Both axes are logarithmic. The wavelength ranges from about 30 nm in the extreme UV to 1 m or VHF radio. The green band marks visible light.

A higher temperature means a higher surface brightness at all wavelengths – a hotter star is brighter, whichever filter we use. A higher temperature also means a shift into the blue – a hot star appears blue, a cool star appears red. Within visible light, the brightness difference at blue relative to red or green wavelengths is quite sensitive to the temperature of the Planck spectrum – the colours of stars that we see are a sign of their surface temperatures.

Key is the expression hν/kT, which is the ratio of the energy taken away by one photon compared to the thermal energy kT of any atom, ion or electron at the stellar surface. Where this ratio is roughly unity, the spectral curve has its maximum and turns over from the blue to the red wing. Far from unity, the Planck curve can be approximated. For the red wing the exp(x) function can be approximated as 1+x and we get (Kneubühl 1982a, p.427):

B_ν ≈ (2 kT / c²) · ν²

B_λ ≈ 2 kT c / λ⁴

Note how h, the hallmark of quantisation, has disappeared again. The red wing of the Planck curve can be understood with classical, pre-quantum physics alone. The brightness in the red wing is proportional to the temperature T and rises with a simple power law to shorter wavelengths.

For the blue wing the approximation is simpler, but not as effective. The one in the denominator is insignificant compared to exp(x). Simply removing it gives:

B_ν ≈ (2 h / c²) · ν³ exp(−hν/kT)

B_λ ≈ (2 h c²) · λ⁻⁵ exp(−hc/λkT)

While the power of the wavelength alone would mean an even steeper rise of brightness with shorter wavelength, the exponential function has the upper hand and causes the steep decline of the Planck curve on the blue wing.

The Planck spectrum has its maximum between the two wings; the wavelength of maximum brightness, or the brightest colour, has a very simple relationship with the temperature of the emitter. This is the Wien displacement law (Tatum 2020a, eq.2.7.1):

λ_max ≈ 2.8978 mm / (T/K)

The brightest colour in the Planck spectrum is directly related to the temperature of the emitter. Say, if deep red (700 nm) is brightest, the star is 4100 K hot at its surface, if deep blue (400 nm) is brightest, the star is 7200 K hot. The Sun at 5800 K should have maximum brightness at 500 nm wavelength.

The black body paradigm allows the star to increase its output when the fusion at its core turns on the heat. The response can be (i) to increase the surface temperature, turn bluer and brighter, and (ii) to increase the size of the star (and hence of the surface). Likely would be a combination of the two.

The black body paradigm has one little problem: It is not true. The assumption is a depth-independent temperature near the surface of the star and an abrupt edge between the stellar surface and empty space outwith the star. In reality, the gas temperature near the surface increases with depth, and above the surface is a less dense corona or atmosphere. These things make the spectrum of a star much more complex and interesting. But the black body paradigm gives us quite good guidance of what is going on.

Open and globular star clusters

Not all stars are "field stars" moving on their own around the centre of the Milky Way. There are also clusterings of stars, and these come in two quite distinct varieties:

Open clusters

These contain each between perhaps 10 and 1000 stars. They appear open in the sense that through the cluster we can see the dark empty space (and some other objects) beyond.

Globular clusters

These each contain tens of thousands of stars. Except for the outskirts, the star density appears very high; we can in general not see through the globular cluster into the space beyond.

Globulars are on average farther away, which contributes to the apparent density. But mainly they are physically different. The number of member stars speaks to this. Globular clusters are bound, only rarely can a star escape the joint gravitational force of the others. Open clusters, while moving through space with a common speed and direction, will over time disperse and disappear into the background of field stars. Further, while open clusters, like field stars, tend to be near the band (or in the disc) of the Milky Way, the globular clusters roam a larger, almost spherical space that encompasses the Milky Way disc. Finally, globular clusters turn out to be old objects with no short-lived blue stars left, while open clusters span the age range with some barely just having started to fuse hydrogen.

Melotte 25 (Hyades) and M45 (Pleiades).


M35 (and NGC2158) and M37.


χ and h Per (Double Cluster); and NGC7789.

Collection of six open star clusters, sorted by distance. Physical parameters:

• Distance: 50 pc, 130 pc, 900 pc, 1.4 kpc, 2.3 kpc, 2.3 kpc
• Apparent radius: 3° to 10'
• Radius: 2.5–10 pc
• Magnitude: 0.5, 1.6, 5.3, 6.2, 3.7/3.8, 6.7

Image parameters:

• Mount: equatorial mount, usually German, usually tracking only
• Lens: photo lens; tele lens; usually ED refractor, 80 mm aperture
• Focal length: 50 mm, f/1.8; 200 mm, f/8, usually 450 mm, f/5.6
• Camera: Canon EOS 300D, but usually Canon EOS 600Dα
• Field of view: 15–1°
• Filters: optionally light pollution filter, outdoor/Sun white balance
• Exposure: 30 s at 400 ISO, stack of 19 frames; 2.5 min at 1600 ISO, stack of 15 frames; 2.5 min at 400 ISO, stack of 8 frames; 45 s at 1600 ISO, stack of 34 frames; 4 min at 1600 ISO, stack of 4 frames; 45 s at 1600 ISO, stack of 34 frames
• Processing: background subtraction, curve stretch
• Location: mostly Edinburgh; more recently Osterholz-Scharmbeck, Germany

M13 and M22.

Two globular clusters. Physical parameters:

• Distance: 6.8 and 3 kpc
• Apparent radius: 12' and 9'
• Radius: 25 and 15 pc
• Magnitude: 5.8 and 5.1

Image parameters:

• Mount: German equatorial mount, tracking, no guiding
• Lens: ED refractor, 80 mm aperture
• Focal length: 560 mm, f/7
• Camera: Canon EOS 600Dα
• Field of view: 0.5°
• Filters: none, outdoor/Sun white balance
• Exposure: 30 s at 1600 ISO, stacks of 25–30 frames
• Processing: background subtraction, curve stretch
• Location: Osterholz-Scharmbeck, Germany

Both types of cluster present us with collections of stars that are at the same distance, but that have also started nuclear fusion of hydrogen at the same time. This makes them interesting objects to introduce one of the fundamental tools of stellar astronomy and physics, the Hertzsprung Russell diagram (HRD).

Star colours and the HRD

Planck spectra B_λ in relation to B (blue) and V (visual or green) filters.

We have seen that the wavelength at which the bulk of radiation is emitted betrays the temperature of the emitting, opaque surface. Although this black-body idea is not exactly true for stars, it gives a very good idea in many respects. This motivates us to measure the colours of stars by measuring their brightness with two different filters. The graphic illustrates this. In a blue B filter and a visual V filter we can measure that a cool 2500 K star is brighter in V than in B. But a hot 10000 K star is brighter in B than in V. Working in magnitudes we can write the colour index as

B−V = B − V

In 1910, Hertzsprung and Russell invented the HRD or Hertzsprung-Russell diagram, a variation of which astronomers still use on a daily basis. In its modern form the colour magnitude diagram places one dot on the graph for each star. The horizontal position marks the colour, usually B−V, the vertical position marks the magnitude, usually V, adjusted to a standard distance of 10 pc. To the left are the blue stars, to the right the red ones.

HRD of the Hyades and Pleiades (data from Hipparcos, ESA 1997a).

The upper graph shows the HRD of two open star clusters that are not far away from the Sun. Red dots are stars of the Hyades (Melotte 25), blue dots are stars of the Pleiades (M45). The graph is quite remarkable. The stars of each cluster line up along a diagonal where red stars are fainter and blue stars are brighter. Each cluster shows the same trend as the other, where V brightens by 6 mag for every one magnitude increase in blueness B−V.

The vertical separation of the two trend lines is due to the fact that the Pleiades are farther away than the Hyades; their apparent magnitudes are fainter. In the lower graph we have corrected the magnitude of each star to a standard distance of 10 pc, i.e. we plot absolute magnitudes on the vertical axis. This shifts all members of the Pleiades up until the trend lines (nearly) match.

The trend takes an upward bend at negative colour indices, at the blue end, and steepens considerably. This is probably just the mathematics of the Planck spectrum: For very hot and blue stars B−V becomes inefficient as a colour measure and all stars that hot have the same B−V value.

But there is also a difference from one star cluster to the other, and this is stellar physics: The Pleiades have these very bright and blue members, but in the Hyades they are missing. There, nothing is bluer than colour index zero. On the other hand, the Hyades have a handful of stars that deviate from the trend. They are bright and red, 6 mag brighter than the trend line would suggest for their colour.

The trend line is the main sequence. It is not a time sequence; stars do not evolve from dim red to bright blue, nor vice versa. The stars on the main sequence are also called dwarfs. In contrast the bright red stars are called giants. The Planck curve prescribes, that at a particular colour stars radiate a particular amount of light per square metre of surface. If the giants are 6 mag brighter (250 times in flux) then they must have 250 times more surface or 15 times larger radius than the dwarfs of the same colour.

The giants then have reached a different balance between fusion in the interior and radiation from the surface. In fact, they must use a different fusion mechanism than the dwarfs or main sequence stars. Giants, rather than fuse hydrogen into helium at the core, fuse hydrogen in layers further up and fuse helium into carbon at the centre.

Why would these stars do that? Simply because they have run out of hydrogen in the core, the only option there is to fuse the resulting helium. In fact, they are, more or less, the stars that are missing from the blue end of the main sequence. We can now understand much of the Hertzsprung Russel diagram:

1. Newly formed stars at their core fuse hydrogen into helium. Such stars form the main sequence and are the dwarf stars.
2. Massive stars have more self-gravity than low-mass stars. They are hotter at the centre and fuse hydrogen more efficiently. This makes their surface hotter and more blue than low-mass stars.
3. The main sequence then is a sequence from massive, hot, blue stars top-left to faint, cool, red stars of low mass toward the bottom-right.
4. Once hydrogen runs out in the core, stars generate even more energy by fusing helium in the core and hydrogen in a shell further out. These stars are larger and redder. In the HRD they lie to the left of (or above) the main sequence.
5. Because massive, blue main sequence stars are more efficient at hydrogen fusion, they run out of fuel sooner than red, low-mass main sequence stars. Hence the main sequence empties from the bright blue end.

There is an unfortunate verbal shorthand relating to this: "Blue stars are young and red stars are old." This is not literally true. True is the following, more subtle thinking:

• A blue star must be young, because it runs out of fuel soon and dies young.
• A red star has fuel for longer; it may be old or young.
• A collective of same-aged stars must be young, if it includes blue (and red) stars.
• A collective of same-aged stars must be old, if it includes only red stars (has no blue stars).
• How red or blue the collective is, may tell its age.

It then follows, for example, that the Hyades is an older open star cluster (600 Myr) than the Pleiades (100 Myr). This is because there are bright blue stars in the Pleiades, but not in the Hyades. The Hyades are still also a young cluster: 600 Myr is nothing compared to the lifetime of the Sun of 10 Gyr. Globular clusters are in general much older than the open clusters of the Milky Way disc. M13 and M22 are 12 Gyr old.

Multiple and variable stars

If we can speak of an ageing of stars, then there has to be a beginning and an end to each star. We observe most stars during their long period of fusing hydrogen into helium. Before, they must have formed from the interstellar gas and dust. This does not happen one star at a time, but more like one gas cloud at a time. Stars form as open clusters that are not gravitationally bound and later disperse. But many stars then also find themselves among one or more close neighbours that they are gravitationally bound to. These are binary and multiple stars. (In English the term double star covers binary stars and also optical double stars, where two stars just happen to be in the same direction from Earth, but are at very different distances.)

A variable star is simply a star that changes its brightness up and down on a reasonably short time scale of between hours and years. Variable stars are the opposite of hydrogen-fusing, main-sequence stars. Young stars can be obstructed by interstellar and circumstellar dust at irregular intervals. Old stars can blast off such material and obscure themselves for periods of time. More regular variables are perhaps fusing helium or higher elements and cannot reach a stable equilibrium of fusion and emission.

Binary and multiple stars

Multiple star systems often take on a hierarchical appearance: Close pairs orbit each other within years, while two or more such pairs have much wider orbits and take thousands or more years to complete an orbit.

Very close binaries can betray their duplicity in their spectrum, with absorption lines of two different types of star. The stars might also eclipse each other; the term eclipsing binary describes such a system, but is actually understood as a type of variable star.

ζ/80 UMa (Mizar/Alcor).


ε^1,2 Lyr.


β Cyg A/B.

Probably the three most famous multiple stars. The three images, when viewed at full resolution, are of the same angular scale.

ζ UMa (Mizar) and 80 UMa (Alcor) are 12' apart, which can be easily separated with the naked eye. With a telescope, Mizar can be separated into two components, ζ¹ and ζ², which are 14" apart. A further star, HD116798, is not part of the physical system.

ε Lyr is a much harder test for the naked eye. The two components, ε¹ and ε², are 3.5' apart. Each is a binary with just over 2" separation. They form a test for small telescopes and for atmospheric conditions.

β Cyg (Albireo) is famous for the colour difference between the two components, A and B. It is not clear that they are a physical binary star; they have similar distance and may form a very wide binary with very long orbital period. Separated by 35". The brighter A has an amber B−V colour index of +1.1 mag, while the fainter B has a green-blue colour index of −0.1 mag.

Physical parameters:

• Distance: 25, 50 and 130 pc

Image parameters:

• Mount: German equatorial mount, tracking, no guiding
• Lens: Schmidt-Cassegrain reflector, 200 mm aperture
• Focal length: 3500 mm, f/17.5
• Camera: Canon EOS 600Dα
• Field of view: 0.04° to 0.4°
• Filters: CLS-visual light pollution filter, outdoor/Sun white balance
• Exposure: 30 ms at 1600 ISO, single frames
• Processing: curve stretch
• Location: Osterholz-Scharmbeck, Germany

Extrinsic variables

An extrinsic variable changes brightness due to outside influences. A common example are eclipsing binaries where the two components of a binary star move in front of and behind each other. β Persei (Algol) is the most famous and probably first discovered variable star. The regularity of the orbital motion of the binary gives the light curve a very precise predictability. However, an eclipsing binary is usually not just an ordinary pair of stars that orbit each other at a leisurely pace. On the contrary, the geometry of eclipses implies that these variables are likely to be very close binaries. They may be in actual contact with each other, or one larger star may spill material onto the smaller one.

Light curve of β Per (Algol).

Due to the regularity of the orbit of most eclipsing binaries, the time of observation can be converted to the phase, i.e. the time since the most recent major minimum. The graph shows my measurements for β Per taken between 2001 and 2012 with two different methods. A phase difference of one equals the orbital period of 2.867328 d. The eclipse lasts about 10 h. The data and indicative grey line show the main minimum at phase zero and the constant brightness at other times. Not observed is the secondary minimum at phase 0.5, which is less than 0.1 mag deep.

Physical parameters:

• Distance: 28 pc
• Apparent V brightness: 2.12 to 3.4 mag
• Period: 2.867328 d
• Radius: 2.7 R_Sun (Algol Aa1) and 3.5 R_Sun (Algol Aa2)
• Luminosity: 180 L_Sun (Algol Aa1) and 7 L_Sun (Algol Aa2)
• Surface temperature: 13000 K (Algol Aa1) and 4500 K (Algol Aa2)

The two components Aa1 and Aa2 that eclipse each other have similar radius, but very different temperature and, due to the Planck law, very different brightness. In the main eclipse, Aa2 all but covers Aa1, leading to the major drop in brightness. In the secondary eclipse Aa2 virtually disappears, but this has only little impact on the overall brightness of the binary.

In 2011 I compiled some project material that may help you observe Algol (or other variables) yourself.

Much harder to observe is the case where a planet orbiting the star transits in front of it and causes a small loss of brightness. Again, the geometry favours planets to be discovered that are (i) large and (ii) close to the star; these planets are called hot jupiters. Best case, you can expect an eclipse of 2% or 0.02 mag of the total light.

The temporary obstruction can also stem from circumstellar material that passes between Earth and the star on occasion. Such a variable can be irregular. The obstructing material may originate from the star or it may be a remnant from the interstellar material that formed the star.

Light curve of ε Aurigae during its 2009/2011 eclipse.

The graph plots the brightness of the star ε Aurigae against time. Values along the bottom axis are Julian Days (minus 2,400,000 days) with the corresponding years and months indicated along the top axis. Values along the vertical axis are stellar magnitudes relative to one of the comparison stars, η Aurigae. The symbol in the bottom left corner indicates the typical precision of the measurements. The measurements themselves are indicated by red crosses. The grey curve is drawn by hand and eye and should be taken with a pinch of salt.

ε Aurigae is an eclipsing variable. About every 27 years, the main star – a white supergiant 135 times the size of the Sun – is eclipsed by a disc of dust and gas that revolves around a blue main sequence star. The blue star and its disc revolve around the white supergiant in 27 years. The disc only ever covers half the face of the supergiant, resulting in the drop of brightness of 0.8 mag. Even then, the remaining light from the supergiant dominates and the blue star remains invisible.

The light curve shows that in late August or early September 2009 the disc began to move in front of the star. By January or February 2010 it had covered as much of the star as it ever would. In March 2011, the disc began to move off the face of the star. This happened about 50% more rapidly and by early June 2011 the whole star was visible again. Both during ingress and egress, the light curve shows a delay around half way through. Finally, during the time between ingress and egress, the eclipse was not constantly deep. Rather, more light from the supergiant seems to make it to Earth around the centre of the eclipse. The shape of the light curve may tell us something about the structure and movement of the eclipsing disc.

Physical parameters:

• Distance: 600 pc
• Radius: 135 R_Sun
• Brightness: 3.0 to 3.8 mag
• Period: 9890 d
• Temperature: 7800 K

Image parameters:

• Mount: photo tripod
• Tracking: none
• Cameras: Canon EOS 300D or Canon EOS 400D
• Detector: 22 × 15 mm
• Focal length: 135 mm
• Field of view: 6°
• Aperture: f/4
• ISO: 100
• Exposure: 5 s
• Location: Edinburgh
• Processing: Groups of 10 raw frames, photometry in the G channel of each frame, use average brightness.

Pulsating variables

A pulsating variable is the case where the star changes shape or overall size. Most famous are the Cepheid variables. The mass and evolutionary progress of these stars places them in a physical instability. Times when more energy is generated than radiated alternate with times when more energy is radiated than generated. This leads to a cycle of change in size, brightness and surface temperature. The period of this cycle depends mostly on the mass, and hence absolute brightness, of the star. This makes Cepheids tools of distance measurement for not too distant galaxies.

Eruptive variables

Eruptive variables change brightness irregularly or semi-regularly. The causes are occasional loss or gain of material. Gain of material is typical for young stars still on their way to the main sequence of hydrogen burning at their core. Loss of material is more typical for old stars in the giant to hypergiant regions of the HRD.

Flare stars appear spectacular, with rare, large, short-lived increases in brightness. In fact the flares are the same as on the Sun, and of similar brightness. It is just that the stars are small and faint, and a "solar flare" on such a star is a hugely significant change in brightness.

Cataclysmic variables

Cataclysmic variables exhibit a once-in-a-lifetime brightness increase of huge magnitude. In a supernova a massive star or stellar core collapses at the end of nuclear fusion into a neutron star or black hole. The collapse bounces and the star explodes, emitting vast amounts of light and ejecting much of its material into the interstellar medium. For a few weeks the supernova can almost compete with its host galaxy in terms of brightness.

Supernova SN2014J in the galaxy M82.

The image shows a supernova that went off in January 2014 in the galaxy M82. The image was taken 2014-01-23, two days after its discovery and a week before it reached peak brightness of 10.5 mag. M82 has a brightness of 8.4 mag, when there is no supernova around, only six or seven times brighter.

Physical parameters:

• Distance: 3.6 Mpc
• Magnitude: 11.7 mag (SN at discovery), 10.5 mag (SN at maximum), 8.4 mag (M82 without SN)

Image parameters:

• Mount: SkyWatcher HEQ5 Pro
• Tracking: sidereal, no guiding
• Camera: Canon EOS 600Dα
• Detector: 22 × 15 mm
• Focal length: 2000 mm, Celestron 8 Schmidt-Cassegrain
• Field of view: 0.25°
• Aperture: f/10
• ISO: 1600
• Exposure: 8 × 30 s
• Location: Edinburgh
• Processing: Stack 17 raw frames, subtract parabolic background, logarithmic contrast stretch.

A nova is a less dramatic event than a supernova. The star is not destroyed, and the reason for the brightness increase is the onset and sudden increase of nuclear fusion. The trigger is mass spilling over from a larger, but fainter companion star.